The Application of Mathematical Principles in Music

We decided to set this text bilingual, since our native language is German and we are not sure that the translation is correct in all aspects.

Wir haben beschlossen diesen Text zweisprachig einzustellen, da unsere Muttersprache deutsch ist und wir nicht sicher sind , dass die Übersetzung in allen Punkten korrekt ist.

The application of mathematical principles in music

by Christian Pörksen and Harry Schreiber

The decimal system is not very helpful for the subdivision of circles. The Duodezimalsystem with its potential to divide by 2,3,4 and 6 on the other hand almost ideal. The duodecimal system has proven itself for the subdivision of time in 12 hour sections for the structure of the days. With a circular hand like at the clock, this can be mapped well. In the sequel we would like to show that the circle is not sufficient as a mathematical model for the subdivision of the tonal worlds of music.

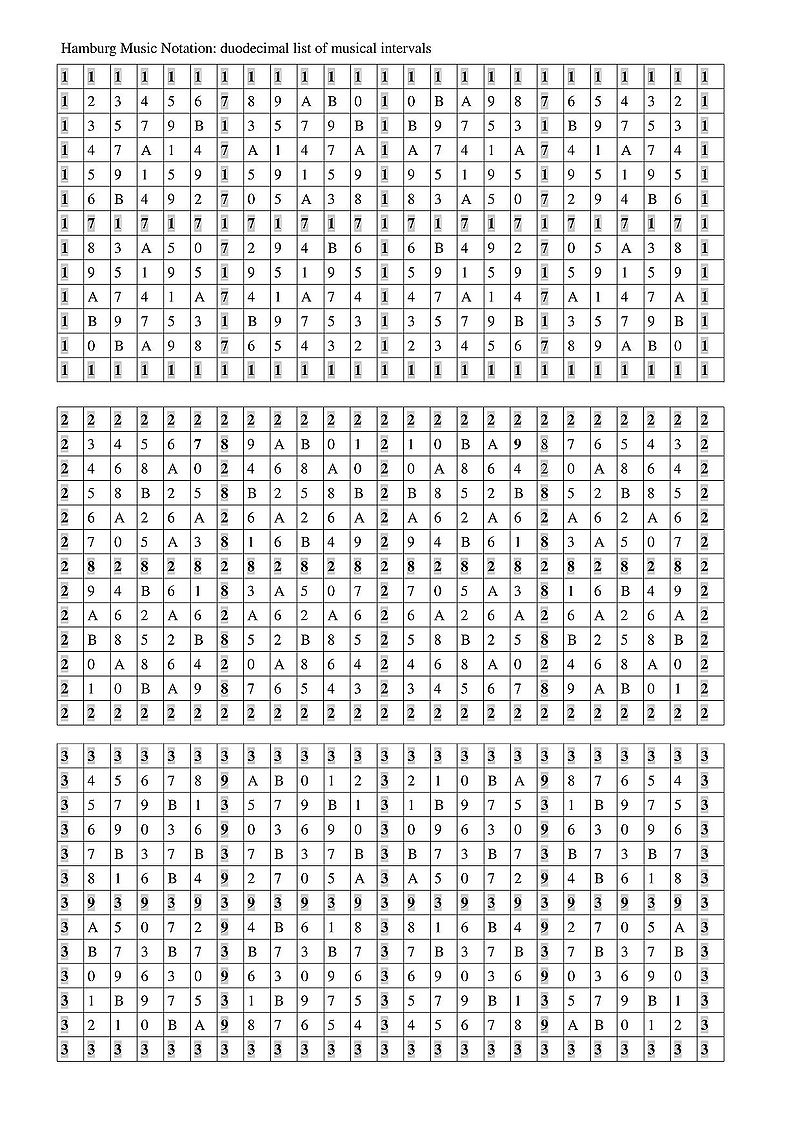
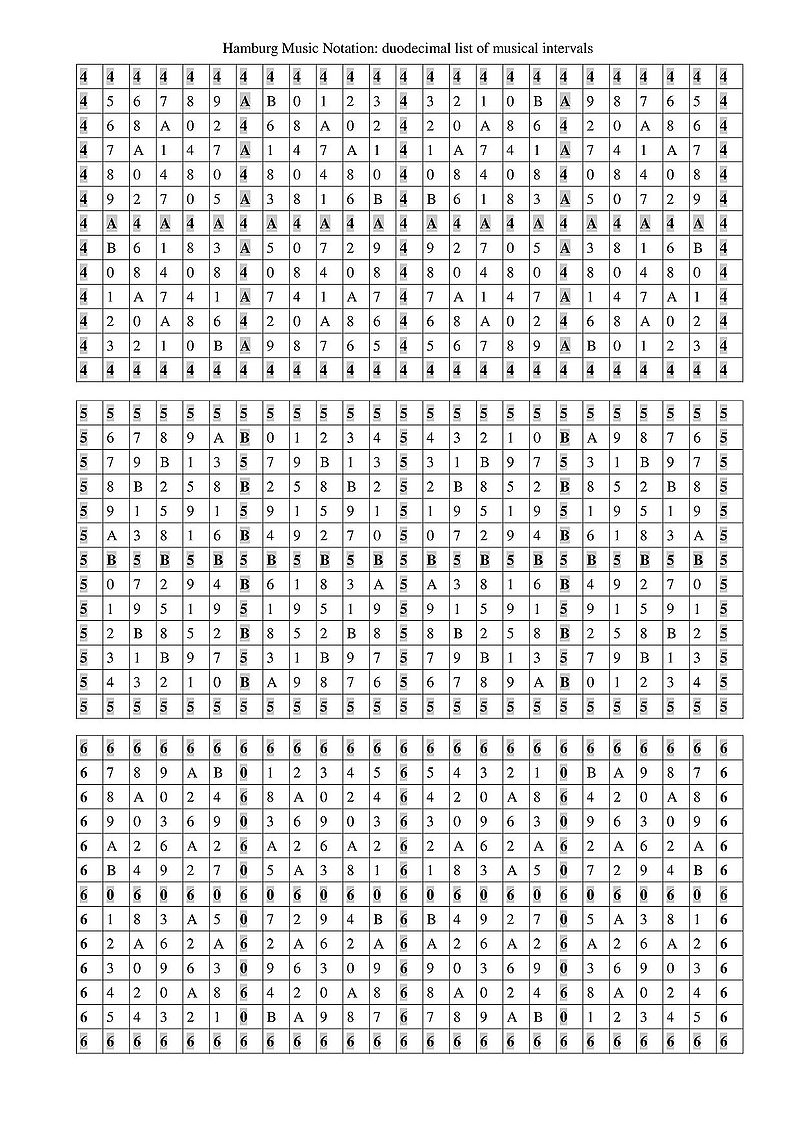
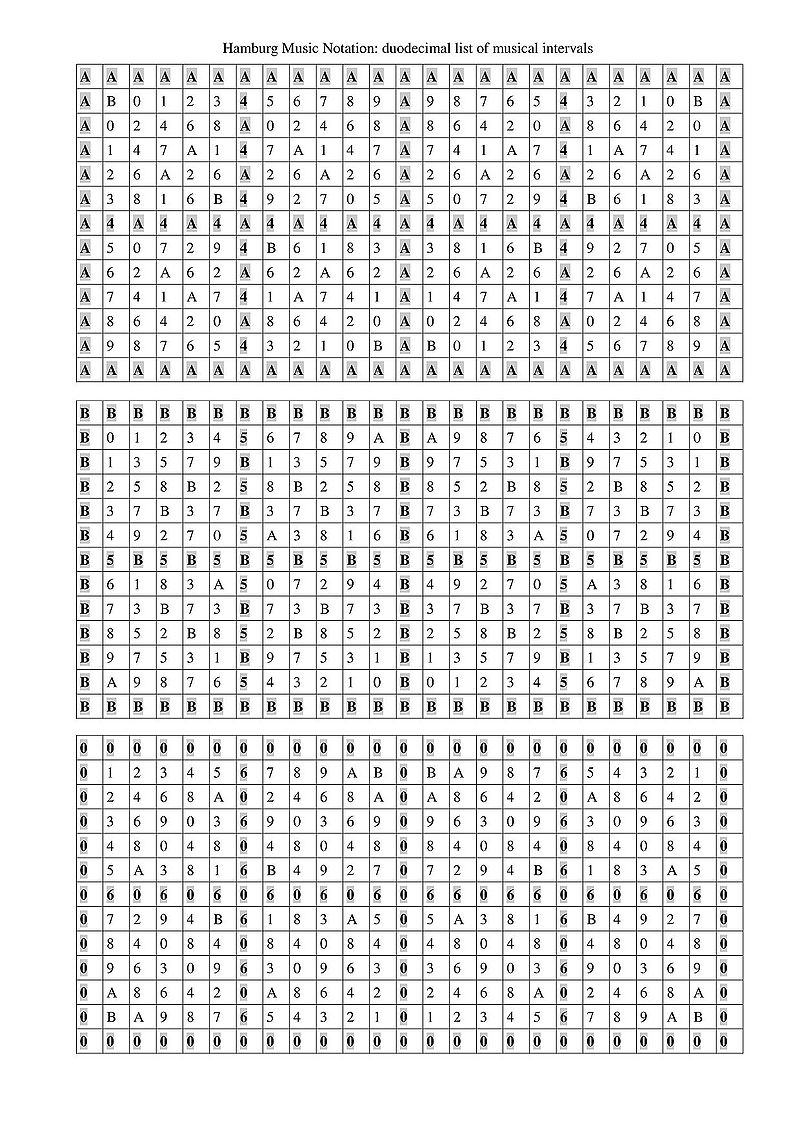
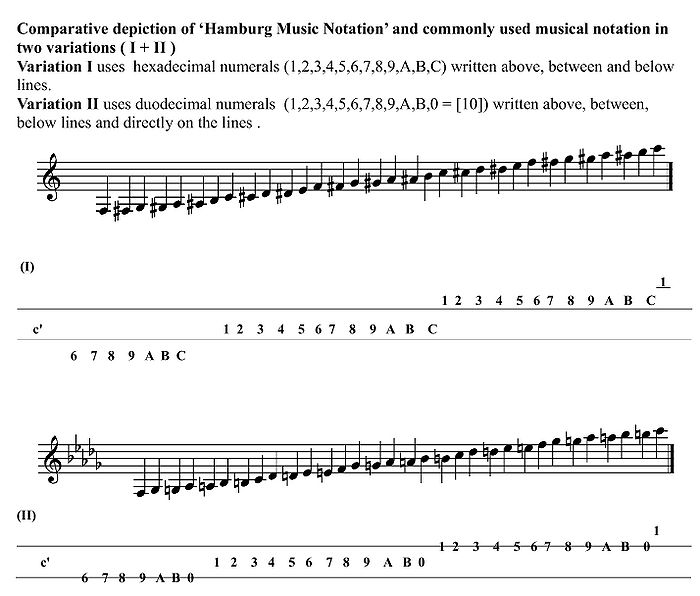
If we start with the C as one, the octave tone c ‘of the higher octave would be the thirteenth tone, or if the duodecimal system is used, the duodecimal eleven. The 13 notes of the twelve pitch steps are given the following ciphers in the Hamburg Musicnotation Version 1705 (HMN1705). (HMN)

1,2,3,4,5,6,7,8,9, A, B, ʘ, separation of the previous octave and beginning of the new octave 1. The letter expansion of our numeral system, which is also used with IBM’s hexadecimal system, is applied.

A for the tenth tone and B for the eleventh tone of decimal counting. For the duodecimal 10 and zero the cipher ʘ is used with a point in the middle. The H, which is designated by a large B in the Anglo-American musical literature, stands at the position of the ten (ʘ) in the duodecimal system. Usually, the sound planes arranged in “octaves” are designated or numbered in different ways. (Eg as by us in the figure 1 duodecimal from 00* to BB* of the Midi table). Midiwerte

One can write each note as a duodecimal number with two digits, e.g. 51 * for the middle C on the piano. The following applies: If the right digit is increased by 1, the next semitone is obtained. If the left digit is increased by 1, the octave of the tone is equal to twice the frequency. The same applies to the subtraction.

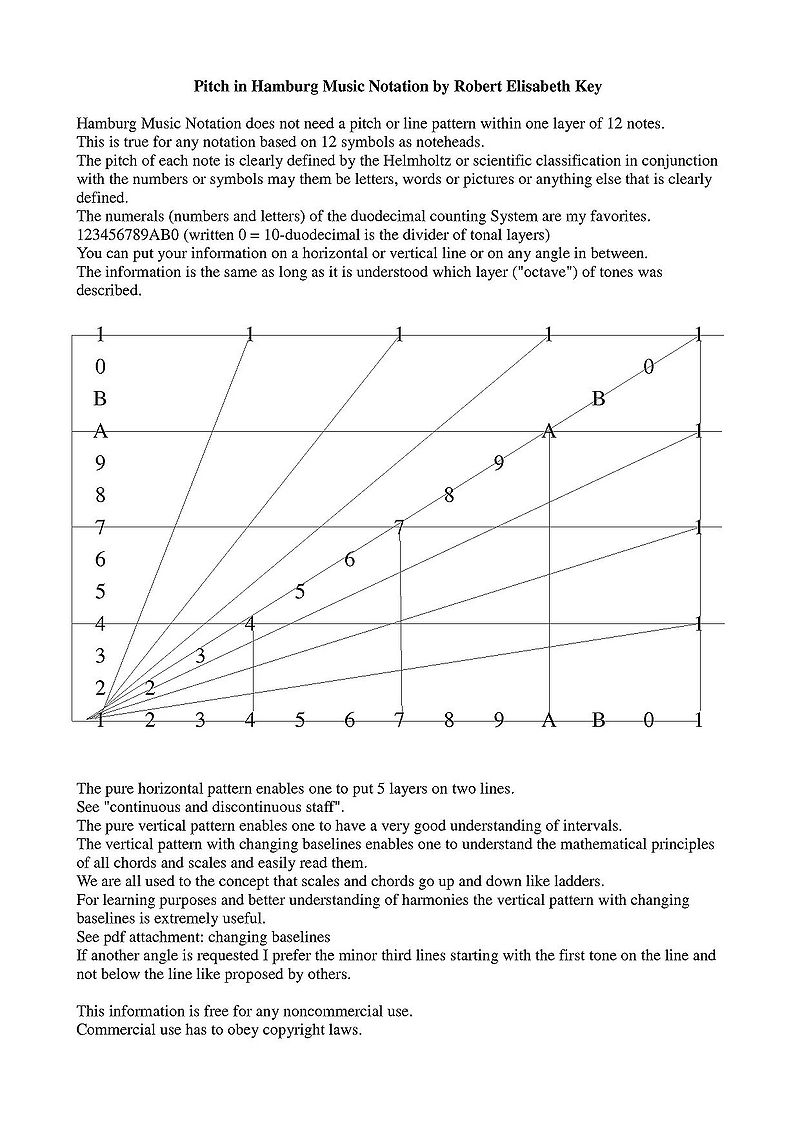
We have decided to name the first note of the octave (= C), as usual, as 1. Then the ʘ is the separator for the next octave. The circle is unsuitable for the correct mathematical representation of the duodecimal system in music, since it does not take into account that the diatonic Western music consists of twelve sound steps, but 13 sounds are required for obtaining 12 steps, which are not identical. This means that after the circle round the same one appears, but actually the one of the next octave is meant.

If one applies the above sequence of numbers to a spiral on plane paper, which has two lines at the same distance, marked at the same intervals as a clock, and then cuts out the zero or tens line along a paper strip, this strip can be applied to a blunt cone And thus gives a very vivid representation of the different sound spaces as the figure 1shows.

Also by means of a ladder (the piano keyboard offers a model for it) all the harmonies of all the scales can be represented upwards and downwards at a clear and correct distance.

A fifths (seven halftones) up from 1=C leads to eight equal to G, and the fifths downwards fom c’ leads to F equal to six. This fact should be given greater attention in the teaching of harmonics and shows the advantages of a mathematical approach. By means of the (ʘ) or (1ʘ) as plane separators, one can move in a circle in any direction by addition or subtraction, but it is necessary to have a plane separator like the ten.

If a fixed value is repeatedly added in a circular scheme, all the numbers in the scheme are obtained if the value of this number is coprime to all numbers in the circle. That means: in the case of 12 numbers, the values are 5,7,11, in this case all primes.

Each tone has its own circles and its own sound planes and this circumstance leads to the almost inexhaustible variety of musical expression.

If the (ʘ) or duodecimal ten (1ʘ) is introduced into the table, which we have already published in 2010 Link, we can move correctly in both directions. See the figure 2 for zero (ʘ) equal to H or B and C equal to one (1).This applies to all other numbers in the same way.

Looking more closely at the above principles, the introduction of a free space above number ten in the HMN 1705 seems to be the key point in simplifying the readability of notations.

A vivid model for the testing of new notations, that meet criterion 8 according to Tom Reed, http://musicnotation.org/systems/criteria/ whose merits and ideas for alternative notations are to be recalled here, can be produced with the table semitones and frequencies from Wikipedia commons.

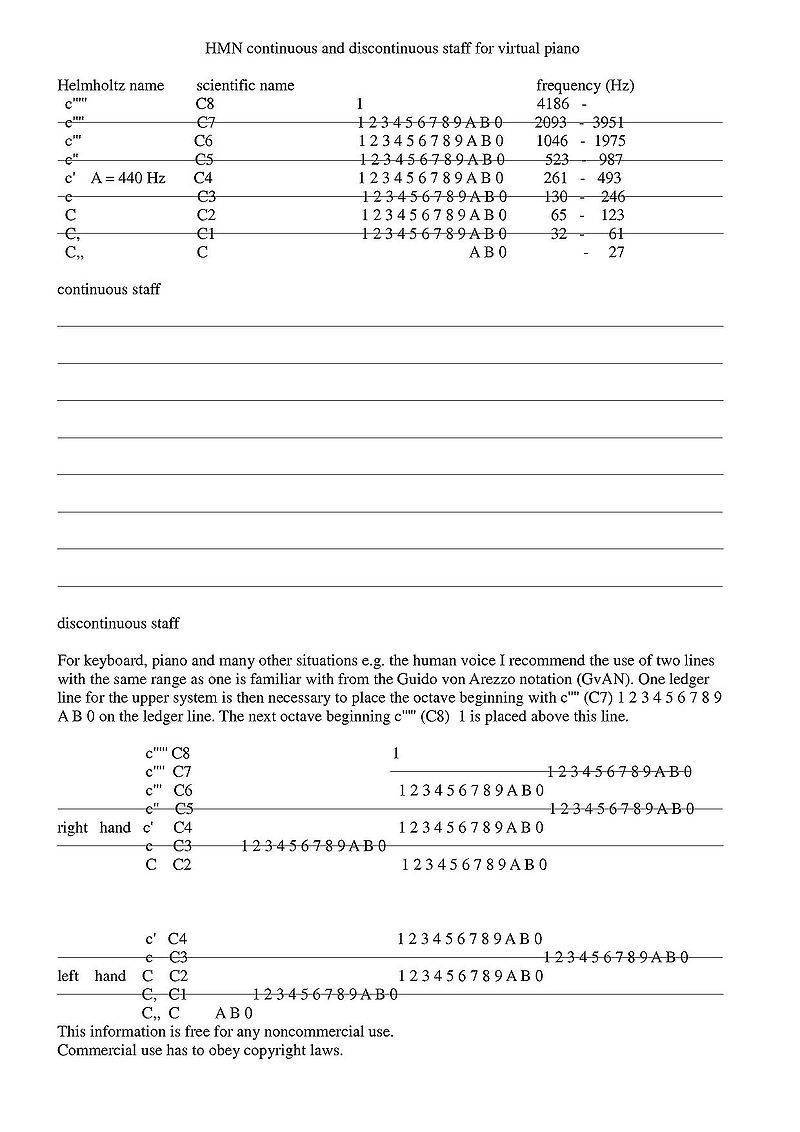
If the table is supplemented by the separator level 10, you can create a cylindrical spiral on which the notes can be mapped ascending and descending as we recently did with the HMN1705. (HMN)

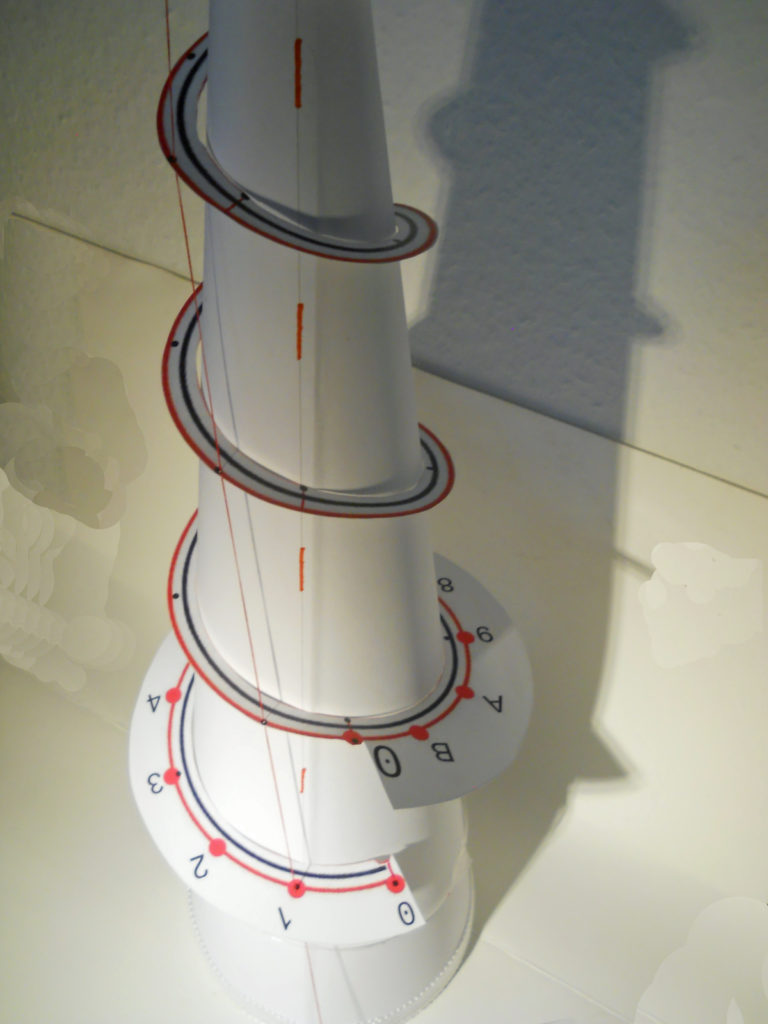
Figure 1 – Abbildung 1

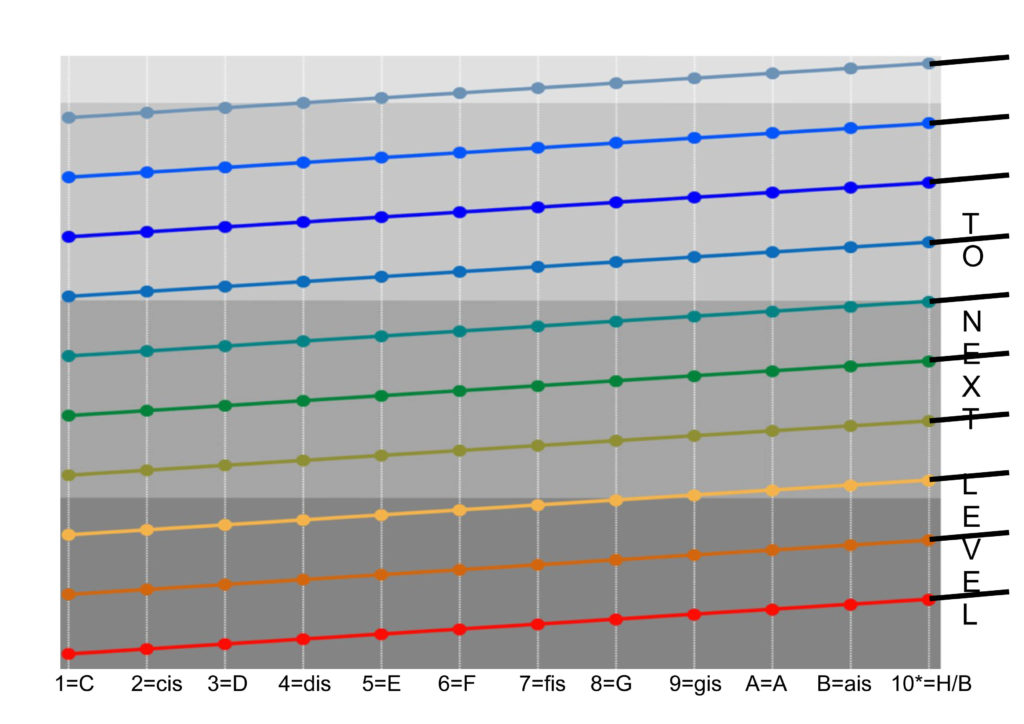
Figure 2 – Abbildung 2

Die Anwendung mathematischer Prinzipien in der Musik

von Christian Pörksen und Harry Schreiber

Das Dezimalsystem ist für die Aufgliederung von Kreisen wenig hilfreich. Das Duodezimalsystem mit seiner Möglichkeit durch 2,3,4 und 6 zu teilen dagegen geradezu ideal. Das Duodezimalsystem hat sich für die Unterteilung der Zeit in 12 Stunden Abschnitte für die Gliederung der Tage bewährt. Mit einem kreisenden Zeiger wie bei der Uhr lässt sich dieses analog gut abbilden. In der Folge möchten wir darstellen dass der Kreis aber als mathematisches Modell zur Untergliederung der Tonwelten in der Musik nicht ausreicht. Falls wir mit dem C als eins beginnen wäre der Oktavton c’ der höheren Oktave der dreizehnte Ton oder bei Anwendung des Duodezimalsystems die duodezimale elf. Die 13 Töne der zwölf Tonschritte werden in der Hamburger Musiknotation Version 1705 (HMN1705) mit folgenden Ziffernbezeichnet. (HMN)

1,2,3,4,5,6,7,8,9,A,B,ʘ, Trennung der vorigen Oktave und Beginn der neuen Oktave 1. Die Buchstabenerweiterung unseres Zahlensystems, die auch beim Hexadezimalsystem von IBM benutzt wird, kommt zur Anwendung. A für den zehnten Ton und B für den elften Ton dezimaler Zählweise. Als Ziffer für die duodezimale 10 und Null wird die ʘ mit einem Punkt in der Mitte benutzt. Das H, welches in der angloamerikanischen Musikliteratur mit einem großen B bezeichnet wird steht auf der Position der Zehn (ʘ) im Duodezimalsystem. Üblicherweise werden die in “Oktaven” gegliederten Klangebenen auf verschiedene Weise bezeichnet oder durchnummeriert. (z. B. Wie von uns in der Abb. 1 Duodezimal von 00* bis BB* der Midi Tabelle angeglichen). Midiwerte

Man kann jede Note als duodezimale Zahl mit zwei Ziffern schreiben, z.B. 51* für das mittlere C auf dem Klavier. Es gilt: Erhöht man jeweils die rechte Ziffer um 1, erhält man den nächsten Halbton. Erhöht man jeweils die linke Ziffer um 1, erreicht man die Oktave des Tons, gleich doppelte Frequenz. Entsprechendes gilt für die Subtraktion.

Wir haben uns entschieden, die erste Note der Oktave (= C), wie meist üblich, mit 1 zu bezeichnen. Dann ist die ʘ der Trenner für die nächste Oktave. Der Kreis ist für die korrekte mathematische Darstellung des Duodezimalsystems in der Musik ungeeignet, da nicht berücksichtigt wird, dass die diatonisch aufgebaute westliche Musik zwar aus zwölf Tonschritten besteht aber für die Erlangung von 12 Schritten 13 Töne benötigt werden, die nicht identisch sind. Das bedeutet, dass nach der Kreisumrundung die gleiche Eins erscheint, aber eigentlich die Eins der nächsten Oktave gemeint ist.

Wenn man die obige Zahlenfolge auf eine in der Fläche dargestellte Spirale, die zwei Linien im gleichen Abstand hat, in gleichen Abständen wie bei einer Uhr aufbringt und anschließend der null bzw. Zehnerlinie entlang einen Papierstreifen ausschneidet, kann man diesen Streifen auf einen stumpfen Kegel aufbringen und erhält so eine sehr anschauliche Darstellung der verschiedenen Klangräume wie die Abbildung 1zeigt.

Auch mittels einer Leiter (Die Pianotastatur bietet sich als Modell dafür an) kann man alle Harmonien aler Tonleitern hinauf und hinab übersichtlich und in korrekten Abständen darstellen. Eine Quinte aufwärts (sieben Halbtöne) trifft vom C gleich eins das G oder die acht und die Quinte abwärts trifft von c’ aus das F gleich 6. Diese Tatsache sollte in der Harmonielehre größere Beachtung finden und zeigt die Vorteile einer mathematischen Betrachtungsweise auf. Mittels der ( ʘ) bzw. ( 1ʘ) als Ebenentrenner kann man sich durch Addition oder Subtraction in korrekten Abständen in jeder Richtung im Kreis bewegen aber es bedarf eben eines Ebenentrenners wie die zehn. Bei wiederholter Addition eines festen Wertes in einem Kreischema, ereicht man genau dann alle Zahlen im Schema, wenn dieser Wert teilerfremd zu der Anzahl der Zahlen im Kreis ist. D.h. Bei 12 Zahlen sind es die Werte 5,7,11, in diesem Fall alles Primzahlen.

Jeder der Töne hat seine eigenen Zirkel und seine eigenen Klangebenen und dieser Umstand führt zu der schier unerschöpflichen Vielfalt musikalischer Ausdrucksmöglichkeiten.

Wenn in die Tabelle, die wir bereits 2010 ins Internet Linkgestellt haben, die ( ʘ) beziehungsweise Duodezimale zehn( 1ʘ) eingeführt wird, können wir uns in beiden Richtungen korrekt bewegen. Siehe Abbildung für die null ( ʘ) gleich H oder B und C gleich eins (1). Dieses gilt für alle anderen Zahlen in gleicher Weise. Abbildung 2

Wenn man die oben genannten Prinzipien genauer betrachtet, scheint die Einführung eines freien Zwischenraumes über der Nummer zehn in der HMN 1705 der Schlüssel zur Vereinfachung der Lesbarkeit von Notationen zu sein.

Ein anschauliches Modell für das ausprobieren von neuen Notationen, die das Kriterium 8 http://musicnotation.org/systems/criteria/ nach Tom Reed erfüllen , an dessen Verdienste und Ideen zu alternativen Notationen hier erinnert werden soll, lässt sich mit der Tabelle Halbtöne und Frequenzen aus Wikipedia commons herstellen.

Wenn die Tabelle durch die Trennebene 10 ergänzt wird, können Sie eine zylindrische Spirale erstellen, auf der die Noten aufsteigend und absteigend abgebildet werden können, wie wir es vor kurzem mit der HMN 1705 gemacht haben. (HMN)




2014

In the meantime we established a partnership with Pizzicato by Arpege Music 
www.arpegemusic.com and developed together the addon Alternative Notation 
http://www.arpegemusic.com/alternative-music-notation.htm
that can handle Hamburg Music Notation and all cipher notations based on twelve 
symbols as well as Numbered Musical Notation (Jianpu). In addition there is a 
vertical pitch version for alternative notations that allows to identify each tone on a 
vertical scale. The software allows to swing back and forthbetween Standard and 
Alternative Notations and can be downloaded for a trial. 
http://www.arpegemusic.com/demo1.htm#go

The very useful manual for Alternative Notation is found on  
http://www.arpegemusic.com/manual36/EN284.htm. 

On  www.hamburgmusicnotation.com you can read on my homepage about theory 
and practice of my Alternative Music Notation System. 

A short ebook with more information is available from AMAZON 
Numbers are Music - Hamburg Music Notation - Jianpu - 
and other Alternative Cipher Notations 
by Robert Elisabeth Key   
Germany: http://www.amazon.de/Numbers-are-Music
UK: http://www.amazon.co.uk/Numbers-are-Music
USA: http://www.amazon.com/usa/Numbers-are-Music